Here are a few noteworthy features of Calcute.
You can enter exact rational values like 2 1\3 instead of a decimal approximation like 2.333333333 that truncates the true value.
You can work in binary, octal and hexadecimal number bases, and not only with integers, but fractional numbers too, even those expressed in scientific notation. You can even mix numbers in different bases within the same expression. For example, use bin'100111 + oct'67 to hex and see the result as hex'5E
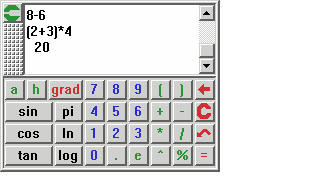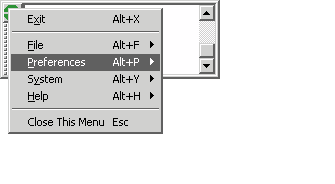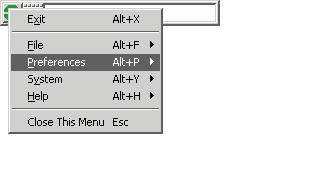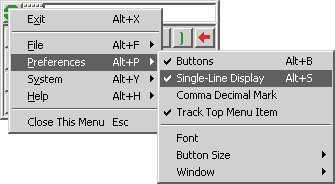
You can make Calcute big or small. The work area is where you enter all calculations and receive results. It can be set to single-line mode to look like a plain calculator. It can be set to multi-line mode for more editing flexibility. You can show the calculator buttons or hide them. Use the Preferences menu options as shown here. Or use the faster equivalent shortcuts: use Alt-S to toggle single-line mode on and off; use Alt-B to toggle calculator buttons on and off.
You can annotate your work. Type anything but hold down the Ctrl key while you press Enter. This way Calcute will not try to evaluate your notes as if they were a mathematical expression so error messages will not be issued for non-numeric text.
You can save your work to a text file, either on request or automatically each time you exit Calcute. The default text file can also reload automatically so you can continue your work where you left off.
You can select any font you like. You can select the button size. You can group digits. You can swap the use of the period and comma to suit your regional preferences.
You can set the calculator window to be always on top. You can pick a preferred screen position where Calcute always opens, or just retain the last position used. Then, use the Alt-D shortcut to dock it back there if it has been moved.
You can change the default hot key used to start the program.
You can be precise. Software arithmetic is used for non-transcendental operations in order to avoid common rounding errors that occur when calculations rely entirely on hardware. This helps to prevent unexpected results, for example very small but non-zero values produced instead of an expected zero result and similar hardware-based errors. Transcendental (logarithmic, trigonometric and hyperbolic) functions remain subject to rounding due to their mathematical nature.
You can get help on all features: a detailed help file is included.